Physics Waves And Oscillations Level: High School
Normal Modes and Resonance Frequencies
A normal mode of a closed system is an oscillation of the system in which all parts oscillate at a single frequency . In general there are an infinite number of such modes . each one with a distinctive frequency f1 and associated pattern of oscillation .Consider an example of a system with normal modes : a string of length L held fixed at both ends , located at x =0 and x =L . Assume that waves on this system propagate with speed v . The string extends in the x direction ,and waves are transverse with displacement along the y direction .A) The string described in the problem introduction is oscillating in one of its normal modes . Which one of the following statement about the wave string is correct ?
a) The wave is traveling in the +x direction
b) The wave is traveling in the –X direction
c) The wave will satisfy the given boundary conditions for any arbitrary wavelength
d) The wavelength have only certain specific values if the boundary conditions are to be satisfied .
B) which of the following statement are true .
a) The system can resonate at only certain resonance frequencies f1 and the wavelength lamda 1 must be such that y1(0,1)=y1(L;t) = 0
b) A1 must be chosen so that wave fits exactly on the string.
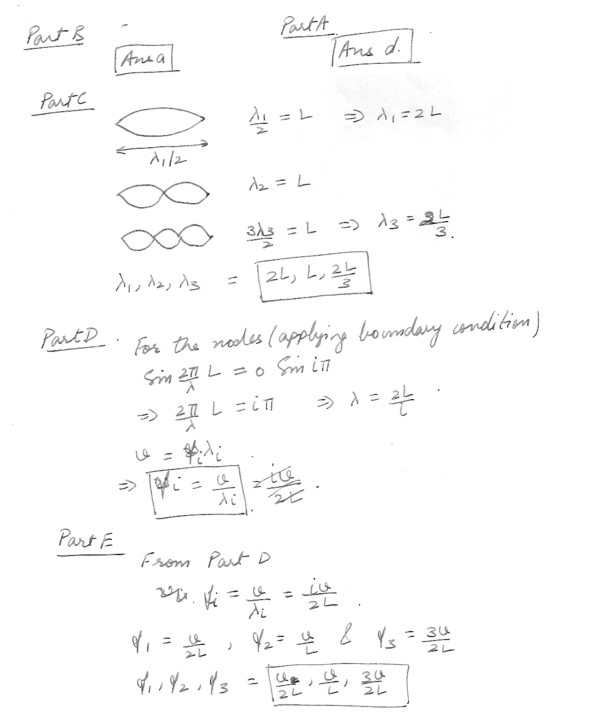
C)Find the wavelengths(call them lamda 1,2,3) that ‘fit’ on the string , that is , those that satisfy the boundary conditions at x = 0 and x = L . These longest wavelengths have the lowest frequencies .
D)The frequency of each normal mode depends on the special part of wave function, which is characterized by its wavelength lamda 1 . Find the frequency f1 of the normal mode