Mathematics Calculus Level: Misc Level
Give a summary of continuity and differential calculus, standard trignometric derivatives, and standard inverse trignometric derivatives.

Mathematics Calculus Level: Misc Level
Give a summary of continuity and differential calculus, standard trignometric derivatives, and standard inverse trignometric derivatives.

Mathematics Calculus Level: Misc Level
find the coordinates of any relative extrema, and classify as maxima or minima.

Mathematics Calculus Level: Misc Level
the annual world rate of water use t years after 1960 was approximately 860e to the.04t power cubic kilometers per year.how much water was used between 1970 and 1980?
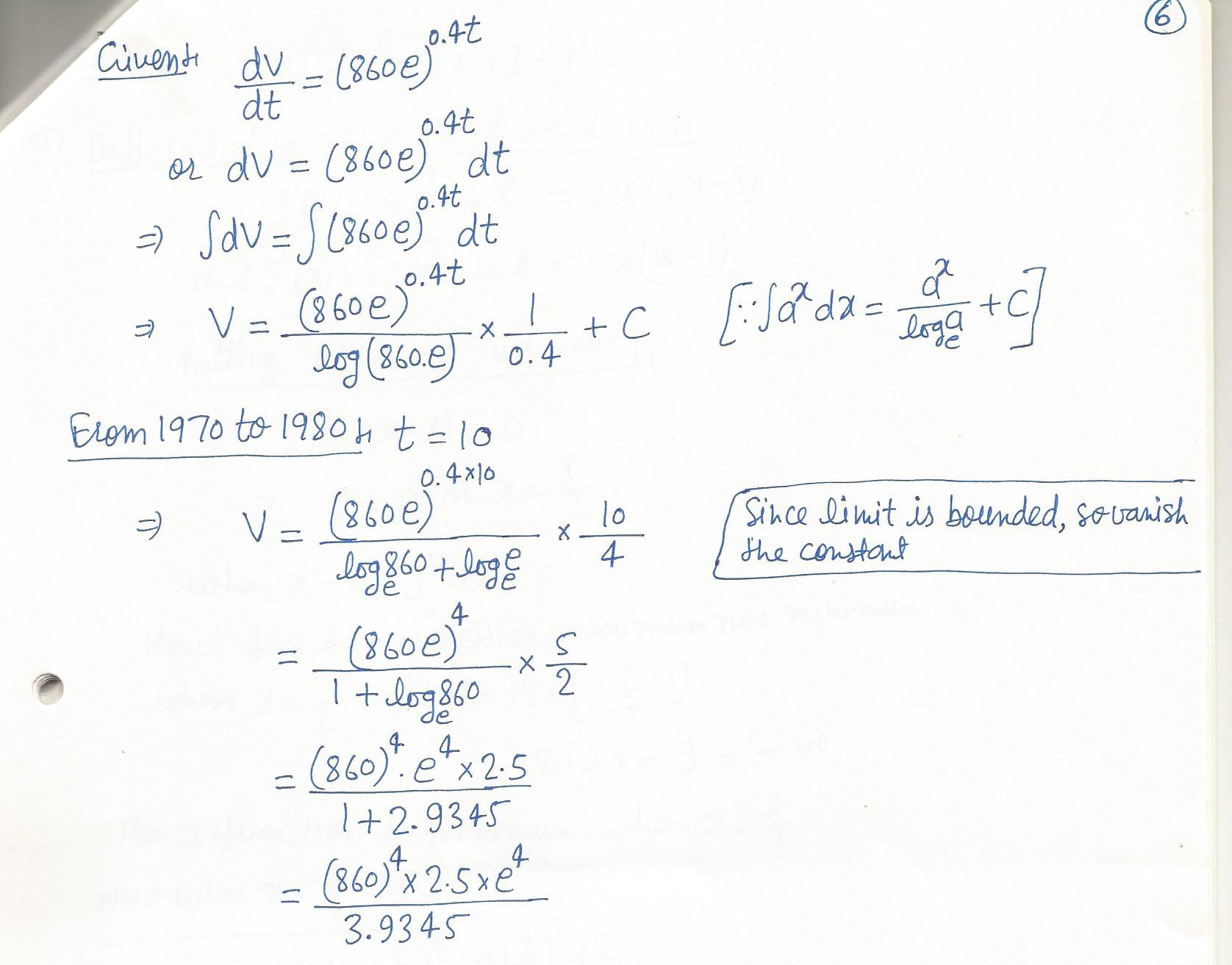
Mathematics Calculus Level: Misc Level
find all relative extrema for f(x,y)=7x2(squared)-5xy+y2(squared)+x-y, and classify each as a maximum or minimum.
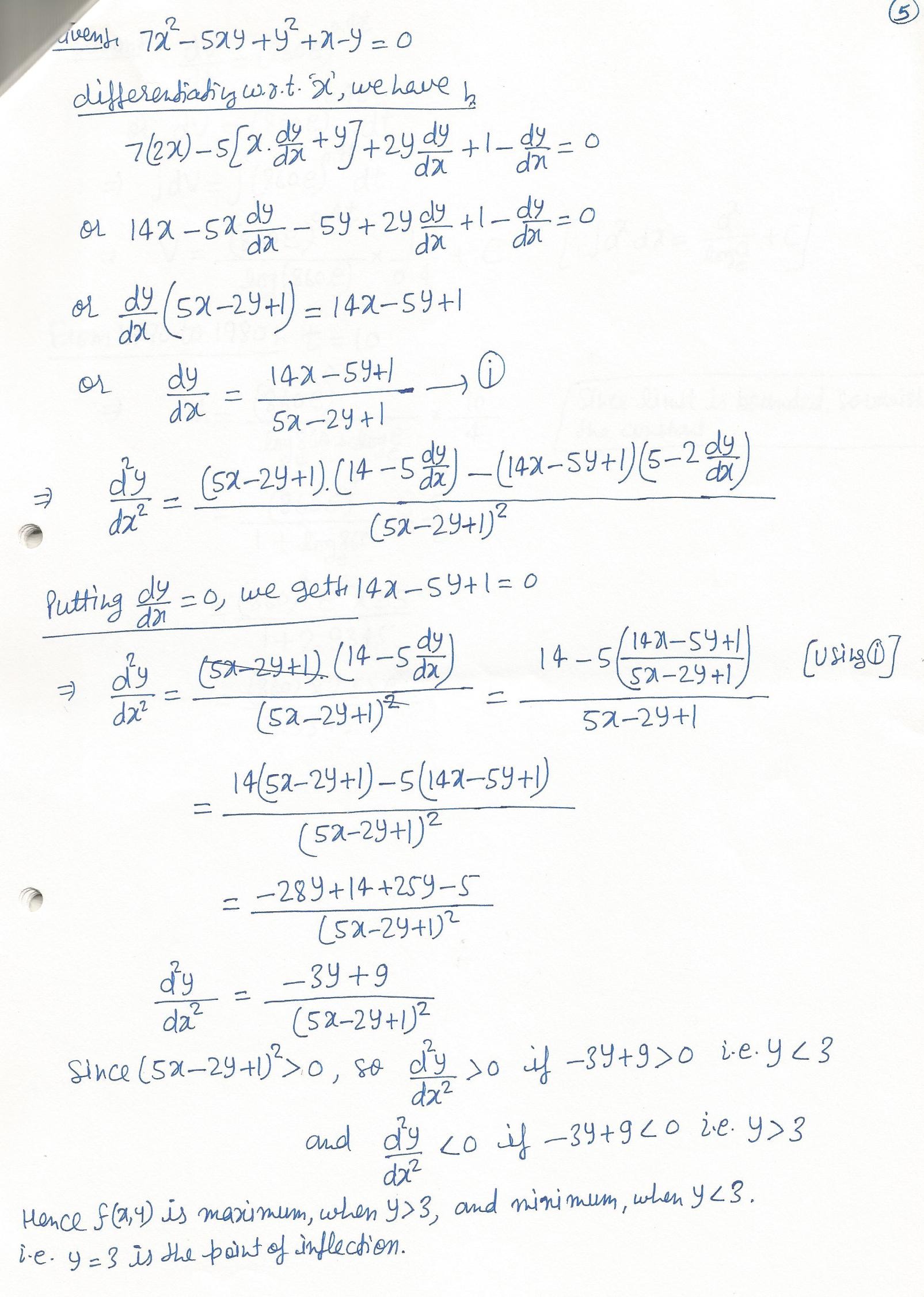
Mathematics Calculus Level: Misc Level
find the volume of the solid bounded by f(x,y)=9-x29squared)-y2(squared) over the region for which 0 is less than or equal to x less than or equal to 2 and 0 is less than or equal to y and is lessthan or equal to 1.
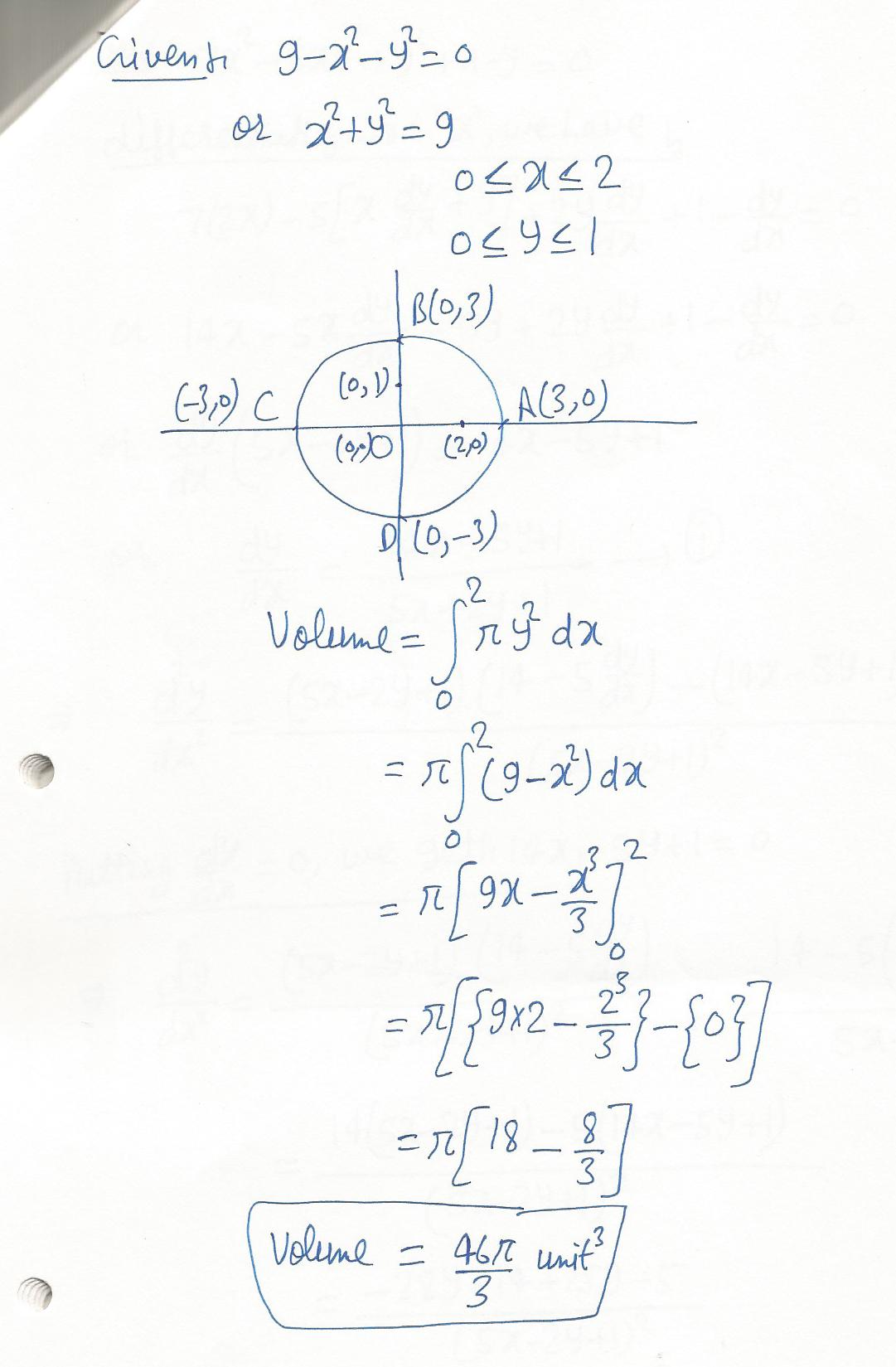
Mathematics Calculus Level: Misc Level
the marginal cost of producing x units of a certain product is C, (x)=6x2 (squared)-4. Find the cost function C(x) if the cost of producing 3 units is $8.
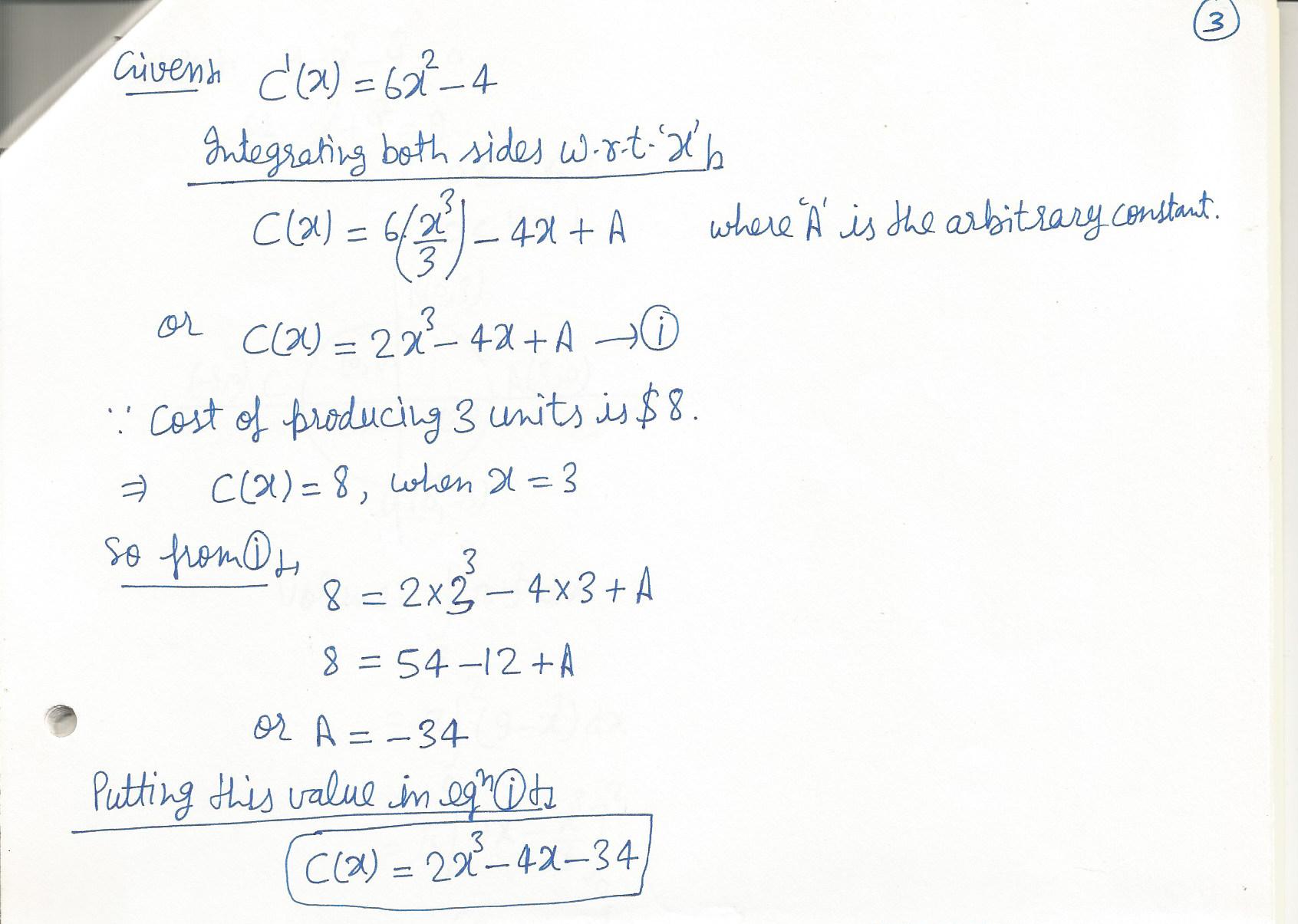
Mathematics Calculus Level: Misc Level
A cylindrical can of radius 5cm and height 12 cm is leaking water at a rate of 4 cm 3 (cubed) per minute. How fast is the height of the water changing when the water level is 3 cm from the bottom of the can.(this is a,,related rates,, problem. Volume of a cylinder is given by V=pie r2(squared)h.)

Mathematics Calculus Level: Misc Level
Consider the function y=f (x)=6x-x2. What is the average value of the portion above the x-axis?First sketch the graph and clearly show the x and y intercepts.
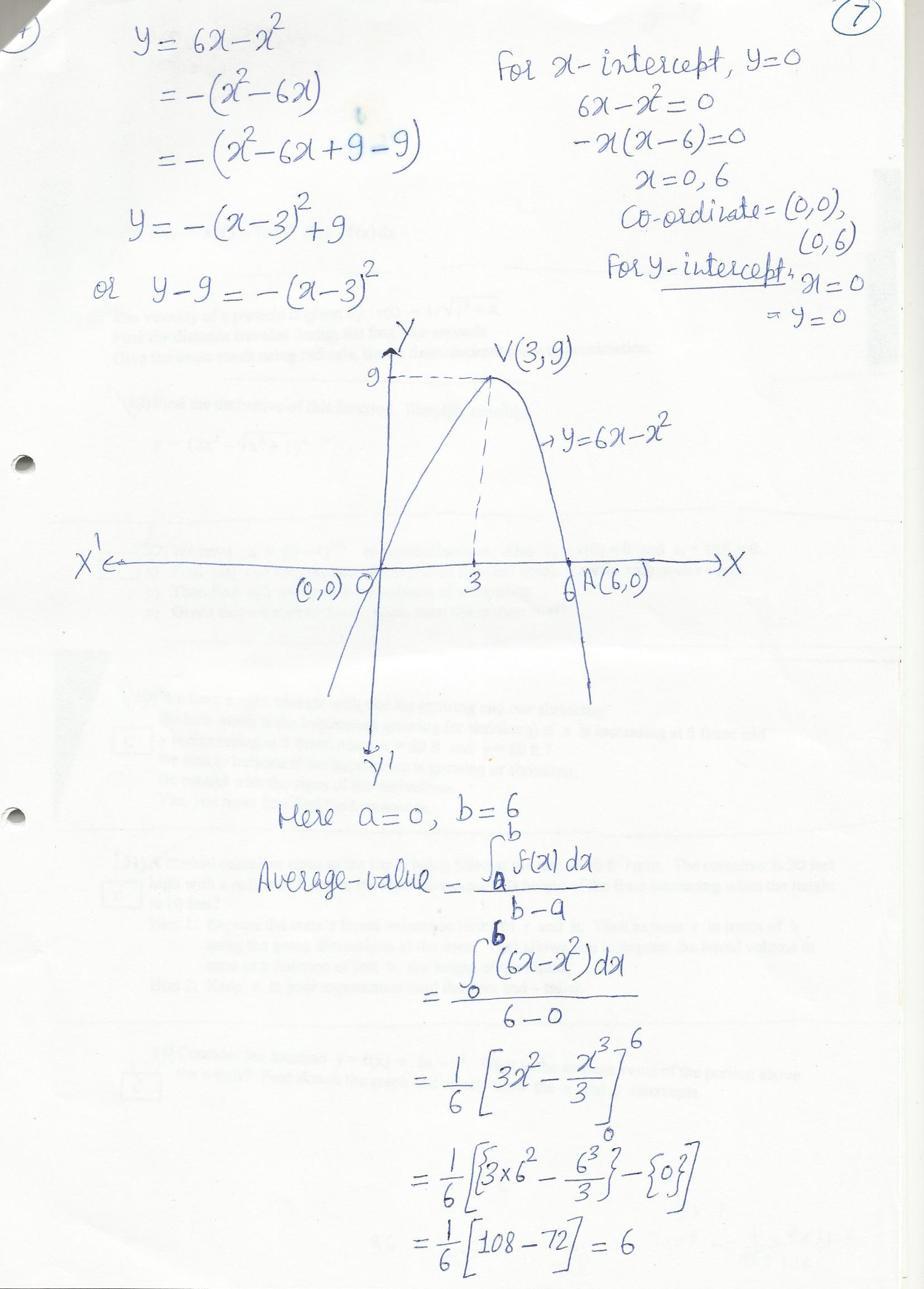
Mathematics Calculus Level: Misc Level
A conical container open to the top is being filled at the rate of 10 ft3/min.The container is 20 feet high with a radius of 6 feet at the top.How rapidly is height of the fluid increasing when the height is 10 feet?
Hint;1; Express the cone,s liquid volume in terms of r and h. Then express r in terms of h using the given dimensions of the cone.That allows you to express the liquid volume in cone as a function of just h, the height of the liquid.
Hint;2;Keep n,in your expressions until the very end-tider.

Mathematics Calculus Level: Misc Level
We have a right triangle with one leg growing and one shrinking.By how much is the hypotenuse growing(or shrinking0 if x is increasing at 6 ft/sec and y is decreasing at 3 ft/sec when x= 60 ft and y=80 ft?Be sure to indicate if the hypotenuse is growing or shrinking.Be careful with the signs of the derivatives.Yes,you must first find the hypotenuse.

Mathematics Calculus Level: Misc Level
The velocity of a particle is given by v(t)=t/(t2+4)^0.5. Find the distance traveled during the first four seconds.Give the exact result using radicals; then a three-decimal place approximation.
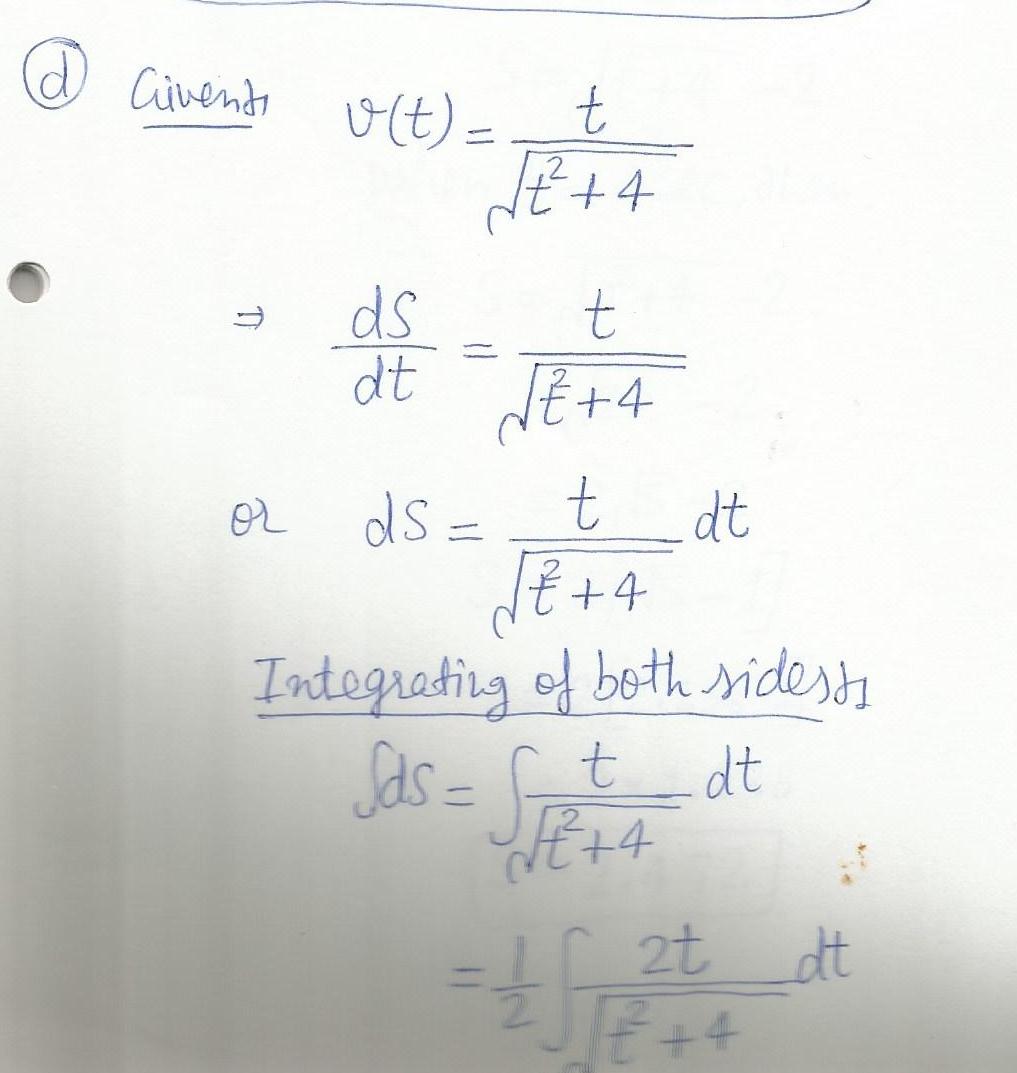
Mathematics Calculus Level: Misc Level
Let f (x)=/x for 1<x <7.Use a Riemann sum withn=6 subintervals and sample points the midpoints of the intervals to estimate the area A between the graph of f and the x-axis. The answer that best describes the estimate is
a. A=13.478
b.A=15.306
c.A=11.680
d.A =11.693
E.None of the other choices is the required Riemann sum
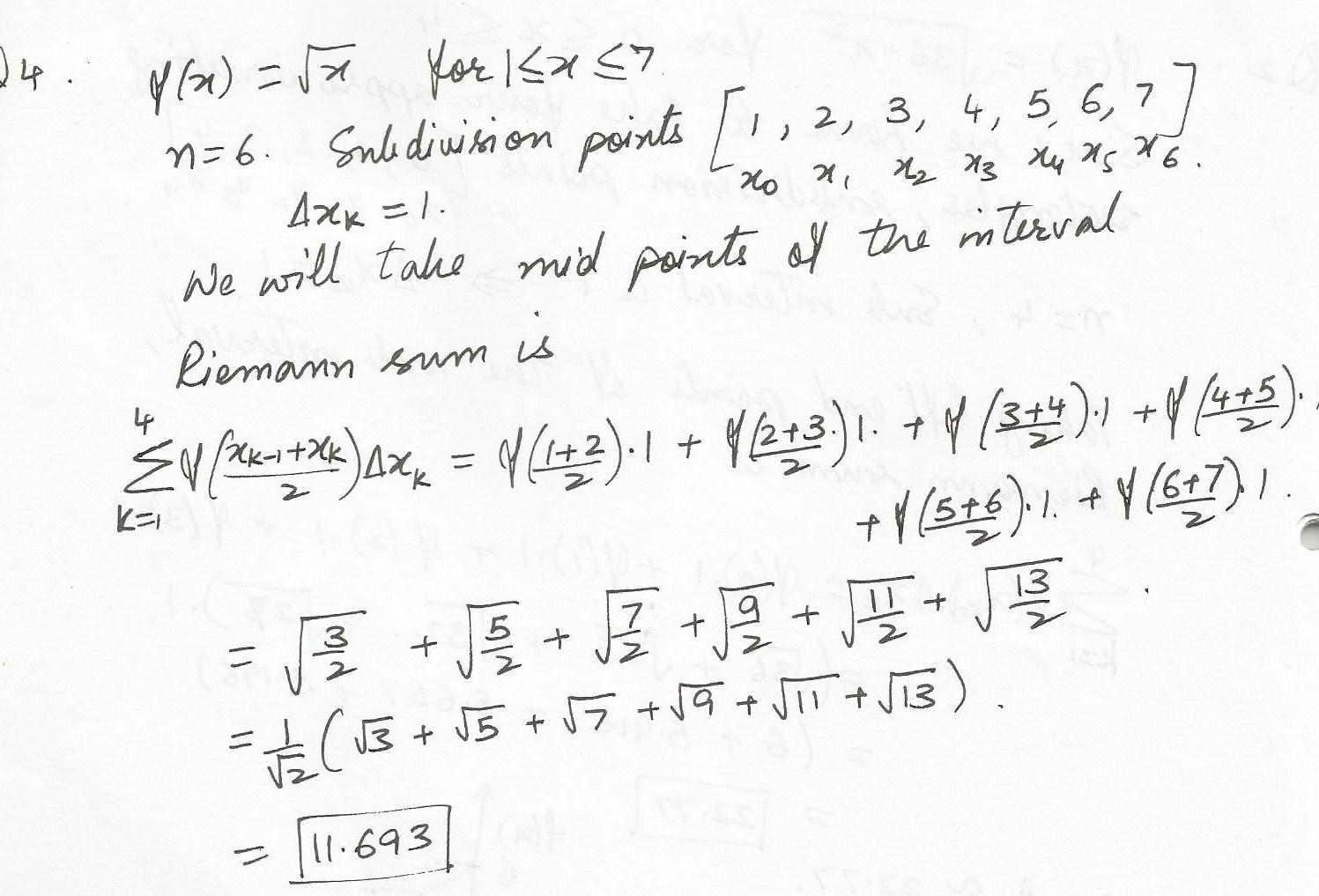
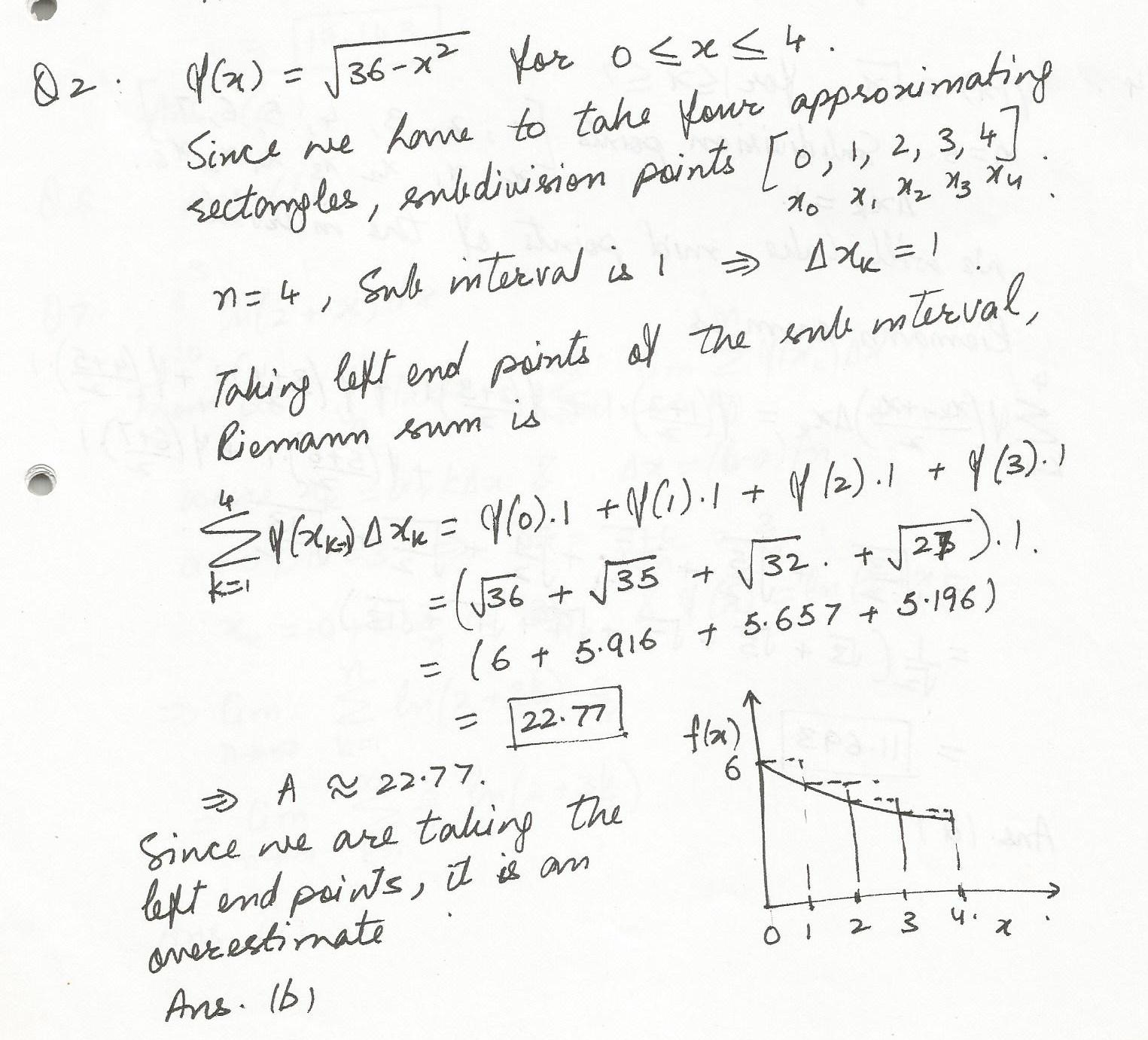
Mathematics Calculus Level: Misc Level
Estimate the area A between the graph f (x)=/36-x2 for 0<4 and the x-axis using four approximating rectangles and left endpoints.The answer that best describes the estimate is
a.A=22.08 which is an under estimate of A.
b. A=22.77 which is an over estimate of A.
c.A=22.08 Which is an over estimate of A.
d.A=22.77 which is an under estimate of A.
e.None of the other choices is correct
Mathematics Calculus Level: Misc Level
Let f (x)=x2 and divide the interval [0,1] into four subintervals determined by the subdivision points 0,1/4,1/2,3/4,1. The Riemann sun of f with sample points the right endpoints of the subintervals is
a.15/16
b.15/32
c.1/3
d.2/3
e.None of the other choices is correct

