Physics Wave Optics Level: Misc Level
The index of refraction of diamond is 2.42. This means that a given wavelength of light travels
2.42 times faster in vacuum than it does in diamond.
2.42 times faster in air than it does in diamond.
2.42 times faster in diamond than it does in vacuum.
2.42 times faster in diamond than it does in air.
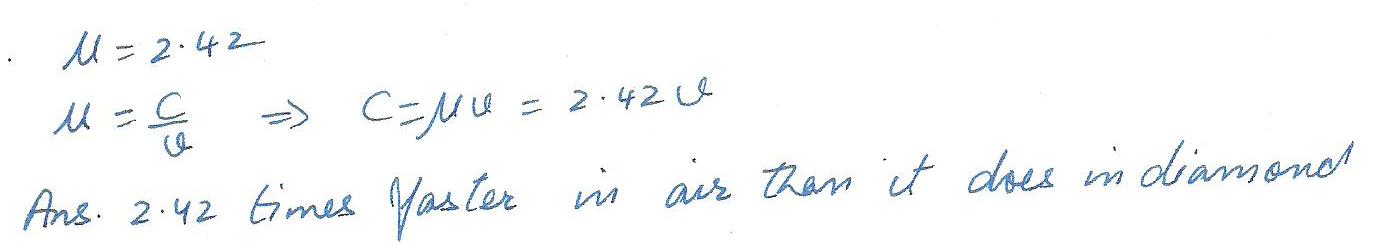
Physics Current Electricity Level: Misc Level
a time delay of 25 ms is required for the voltage to fall to 20% of its maximum value.rearrange the equation to find a new value of resistance to achieve this if the capacitance is kept the same.
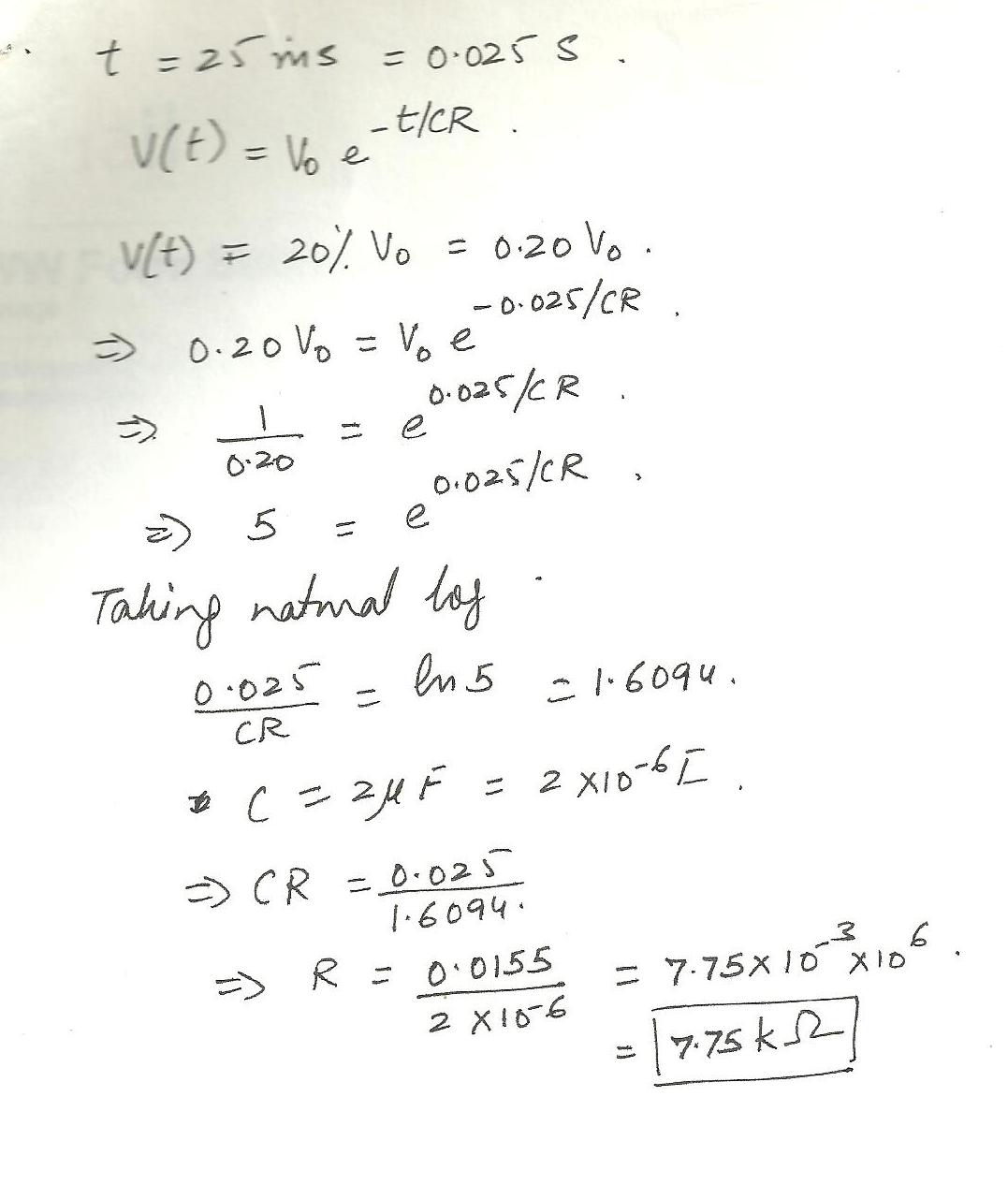
Mathematics Integration Level: Misc Level
Special type
Integrate the following
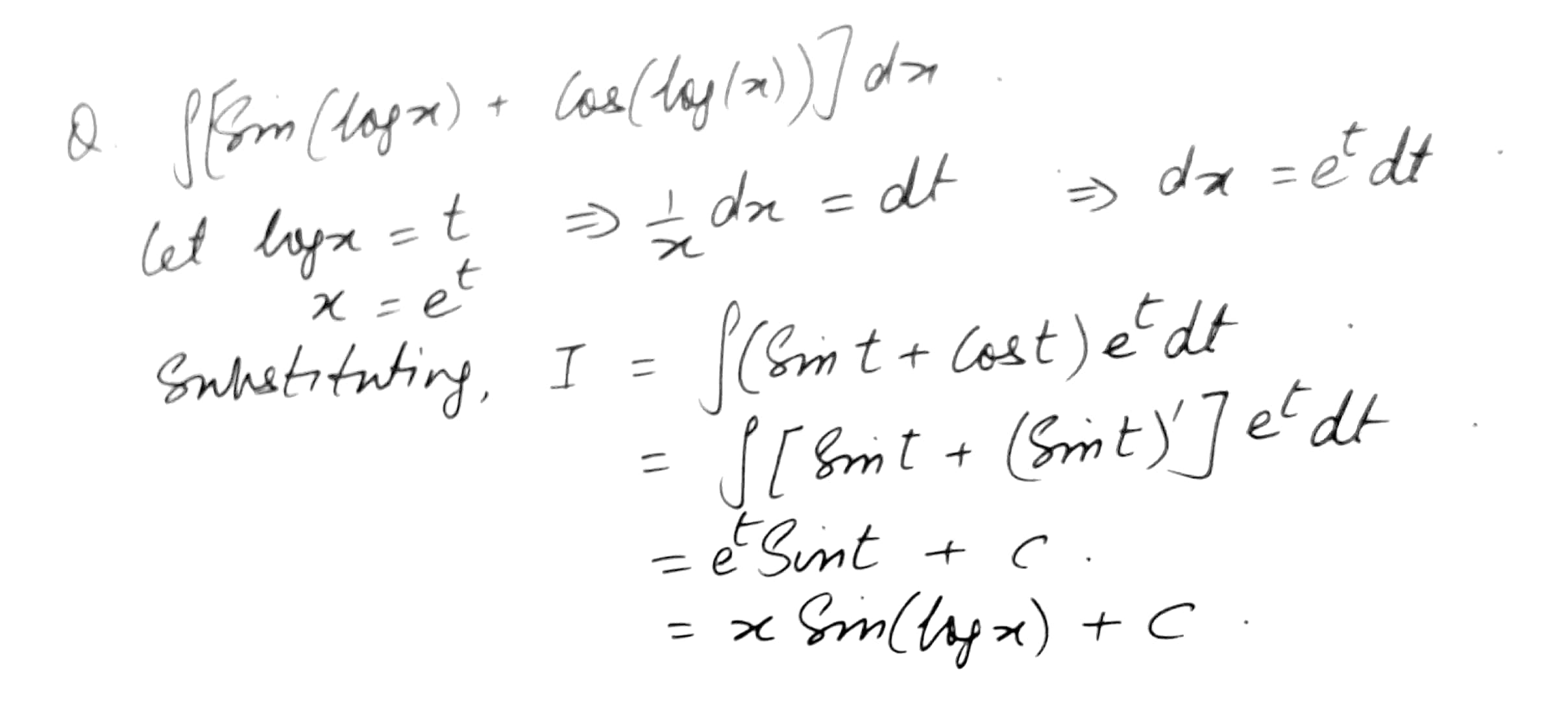
Mathematics Integration Level: Misc Level
Special type
Integrate the following
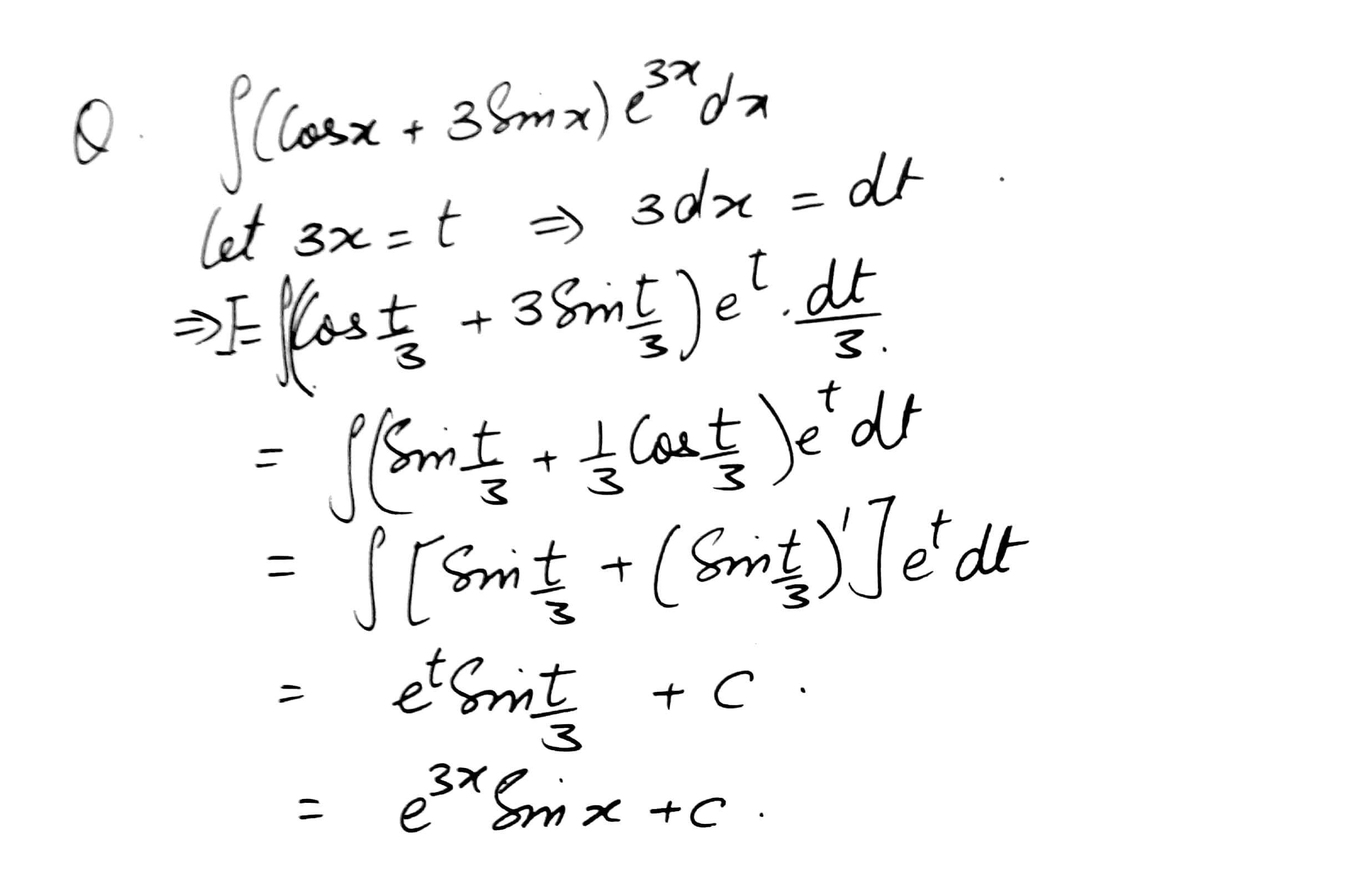
Mathematics Integration Level: Misc Level
Special type
Integrate the following
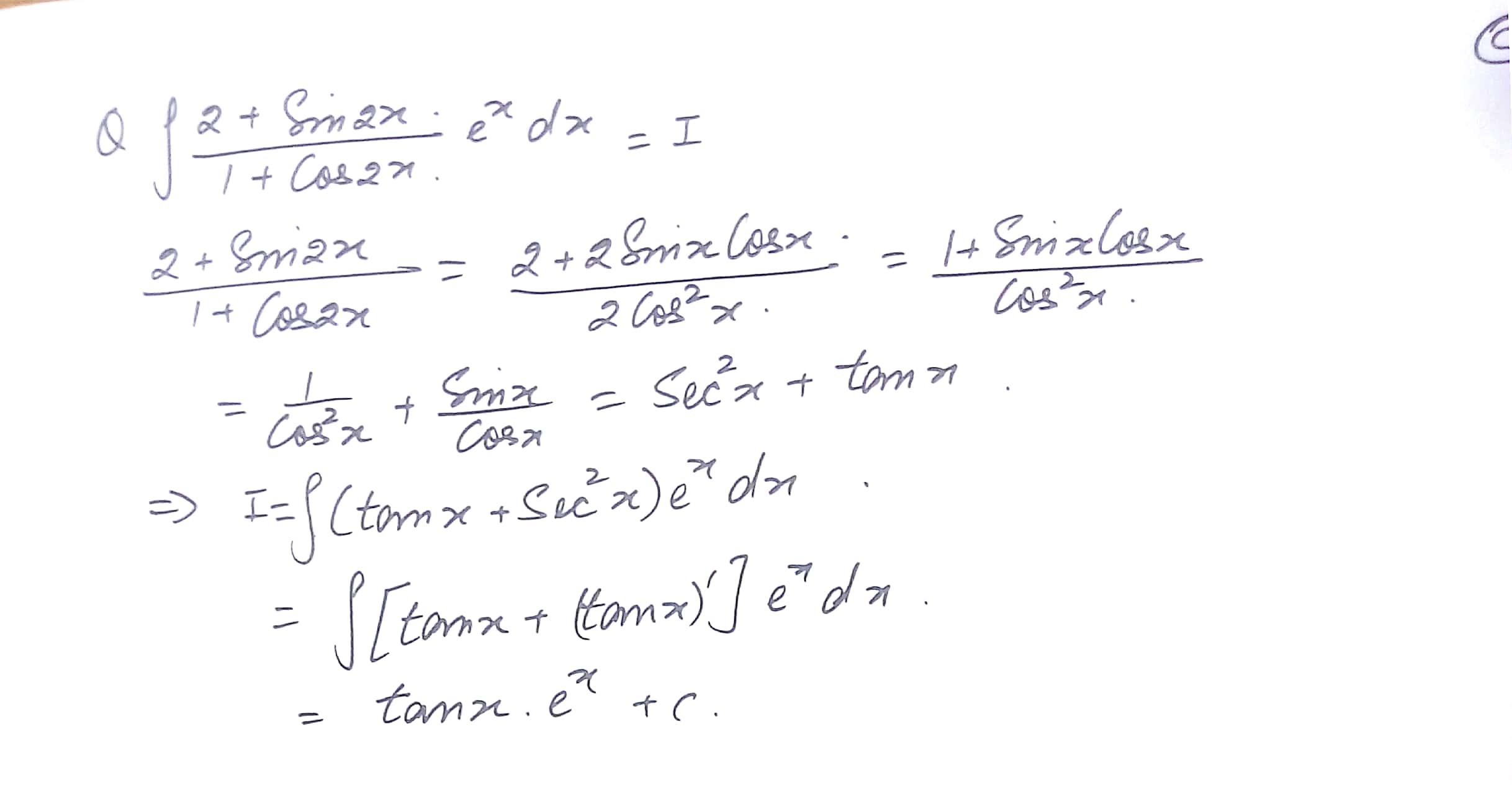
Mathematics Integration Level: Misc Level
Special type
Integrate the following
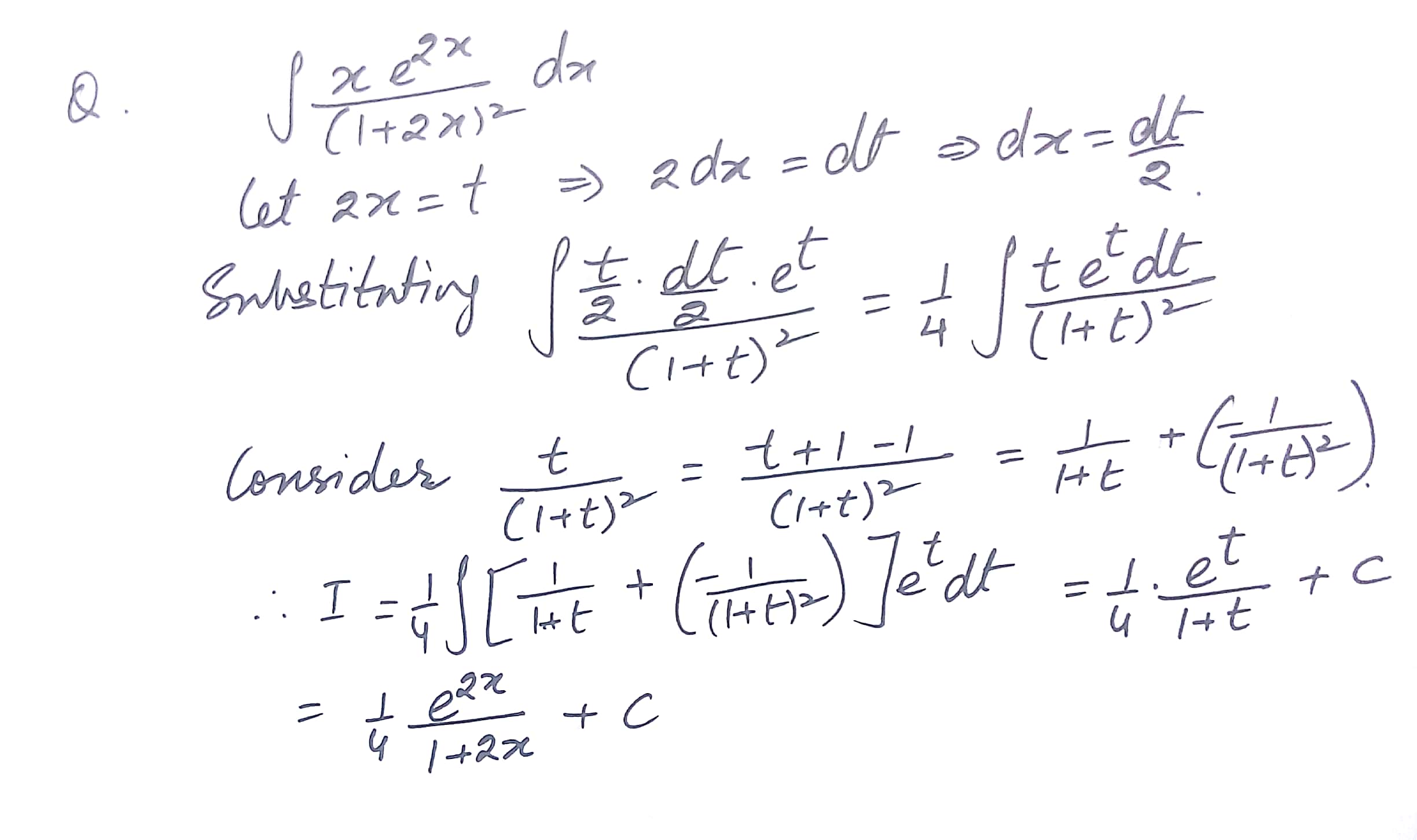
Mathematics Integration Level: Misc Level
Special type
Integrate the following
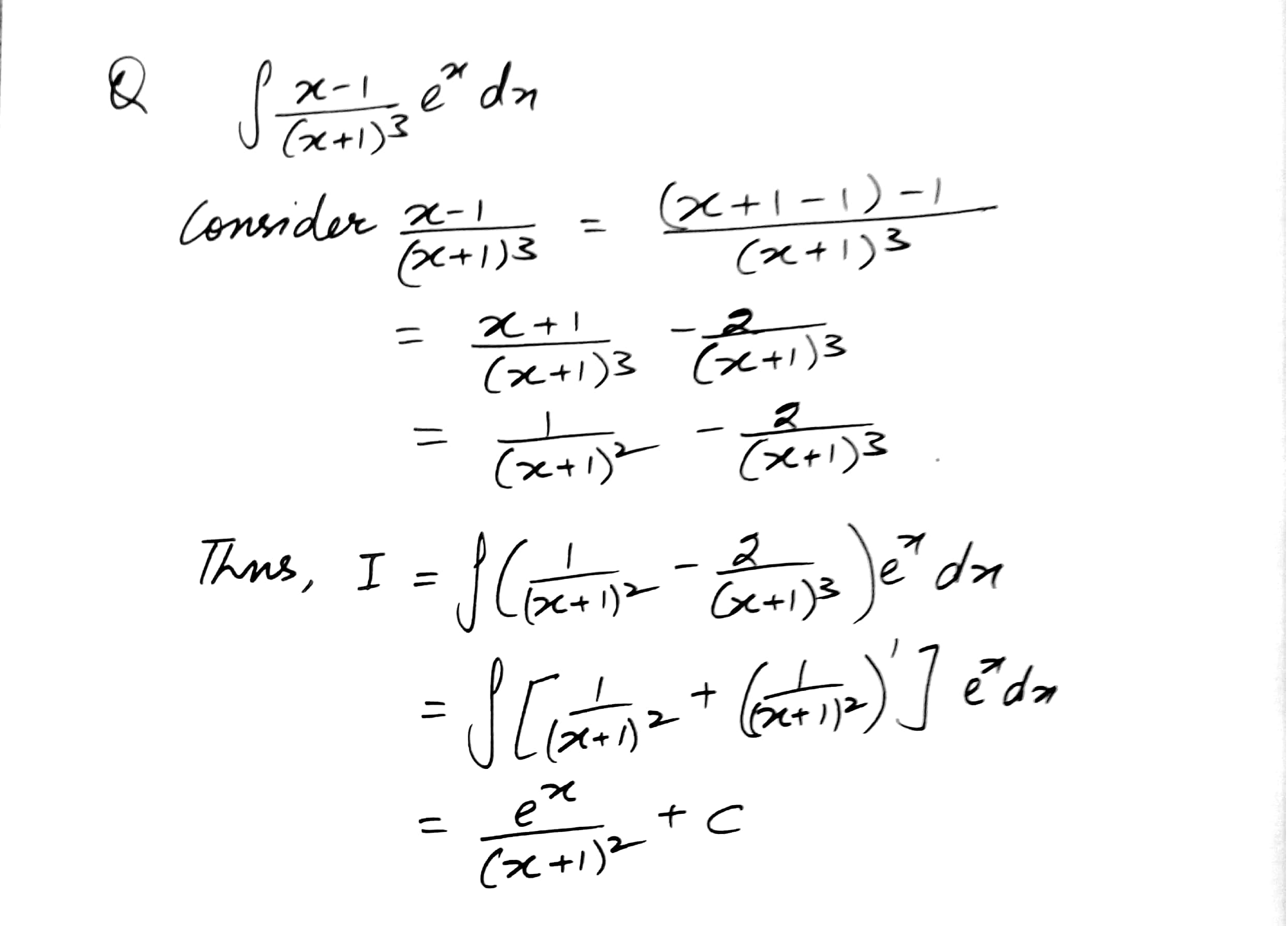
Mathematics Integration Level: Misc Level
Special type
Integrate the following
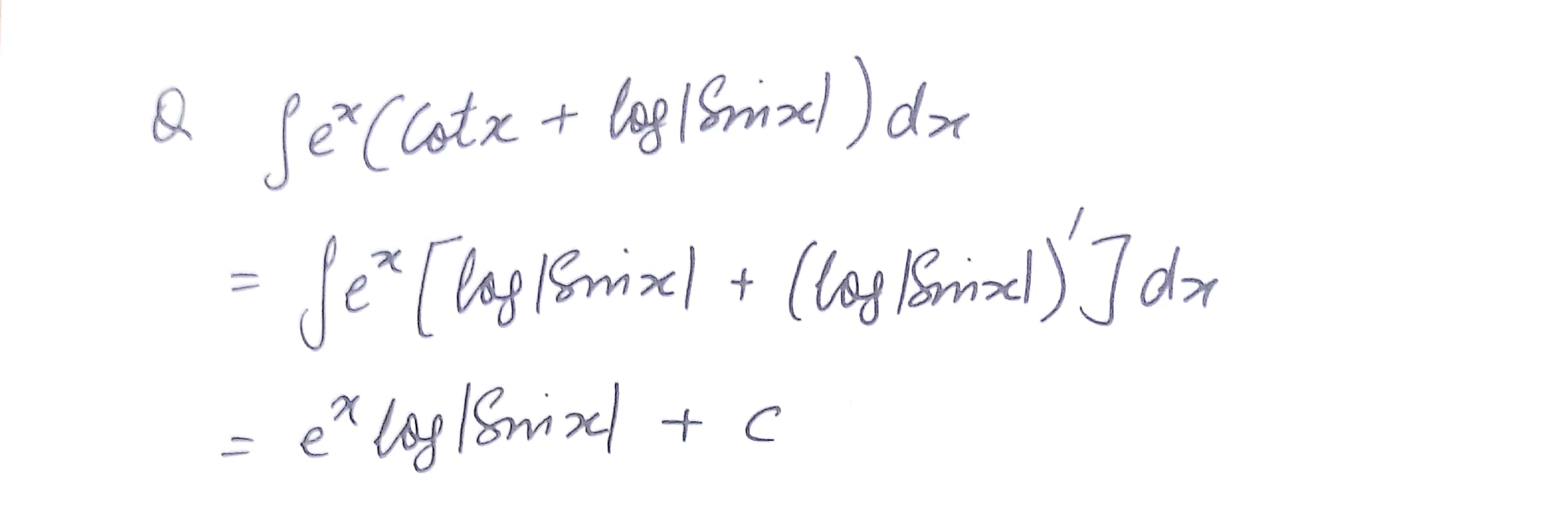
Mathematics Integration Level: Misc Level
Special type
Integrate the following
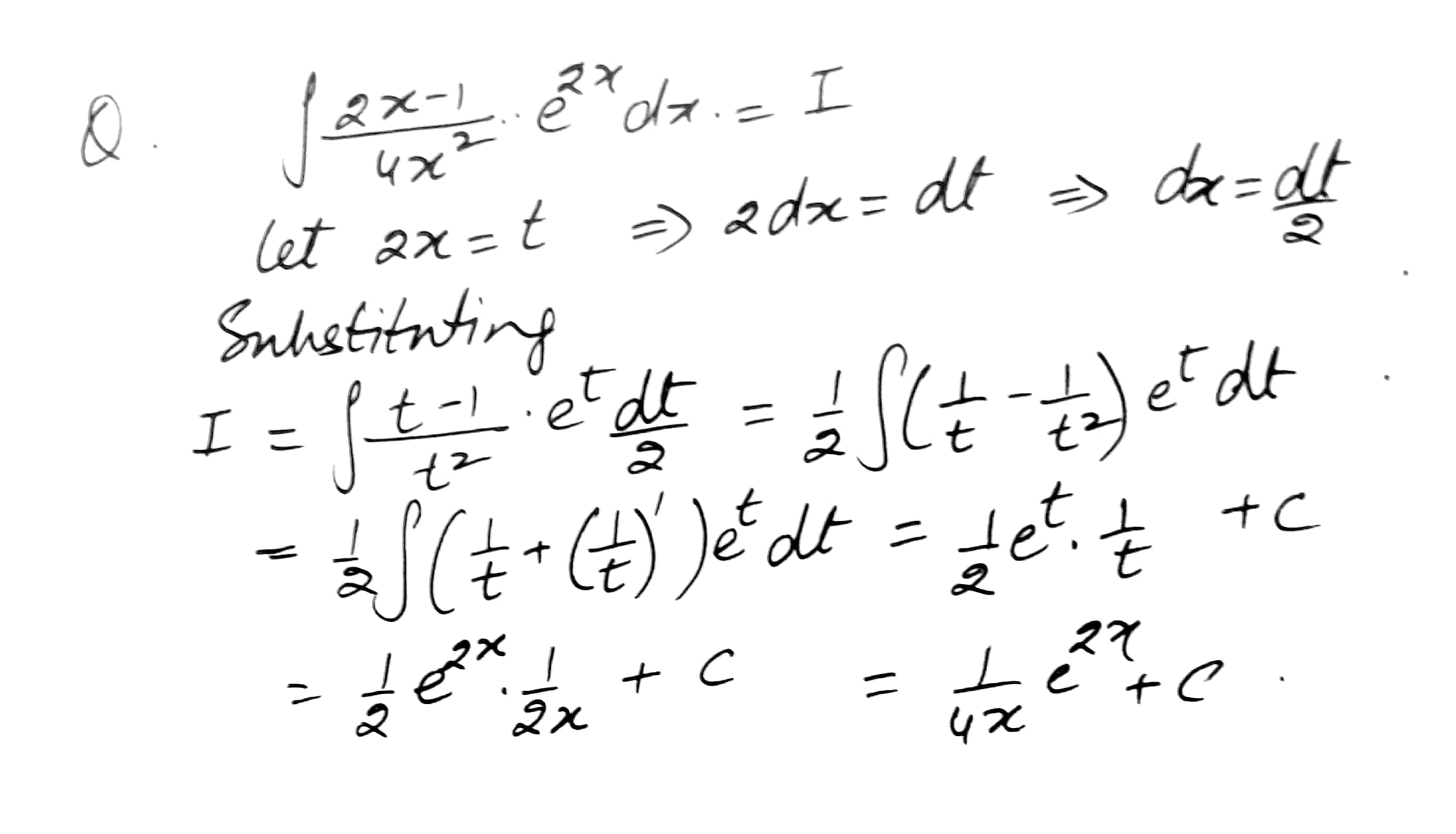
Mathematics Integration Level: Misc Level
Special type
If then find f(x)
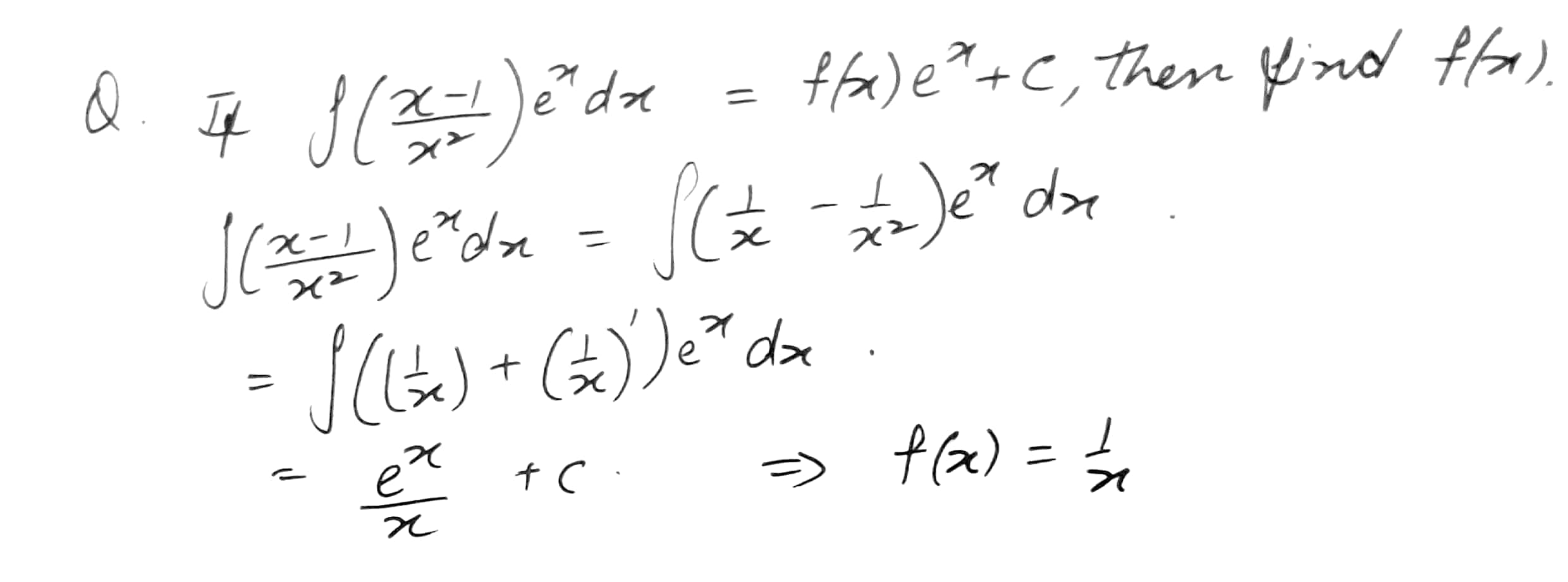
Mathematics Integration Level: Misc Level
Special type
Integrate the following
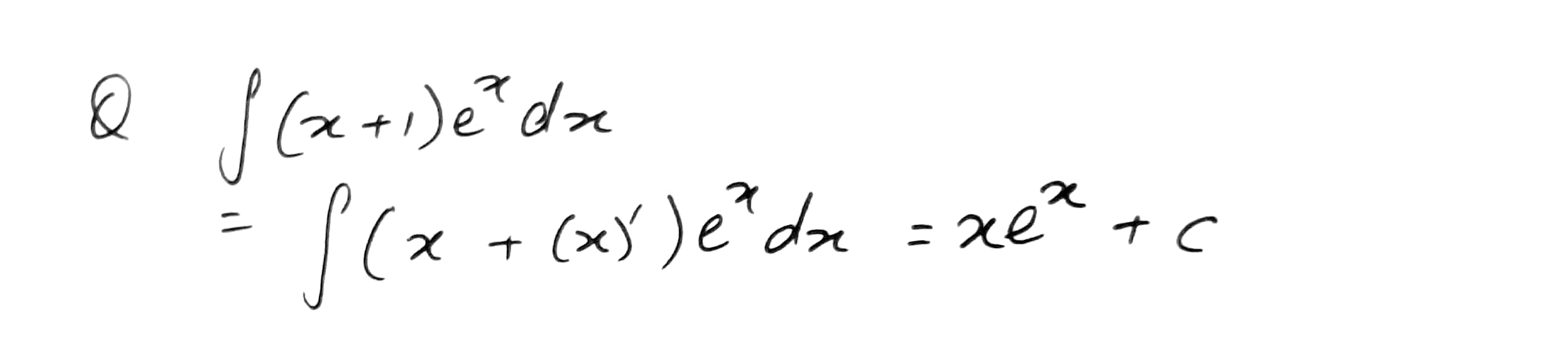
Mathematics Integration Level: Misc Level
Special type
Integrate the following
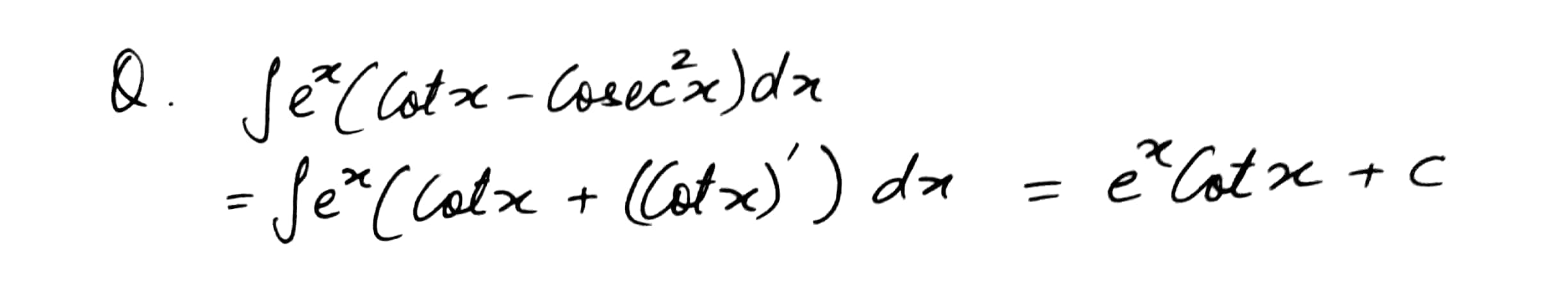
Mathematics Integration Level: Misc Level
Special type
Integrate the following
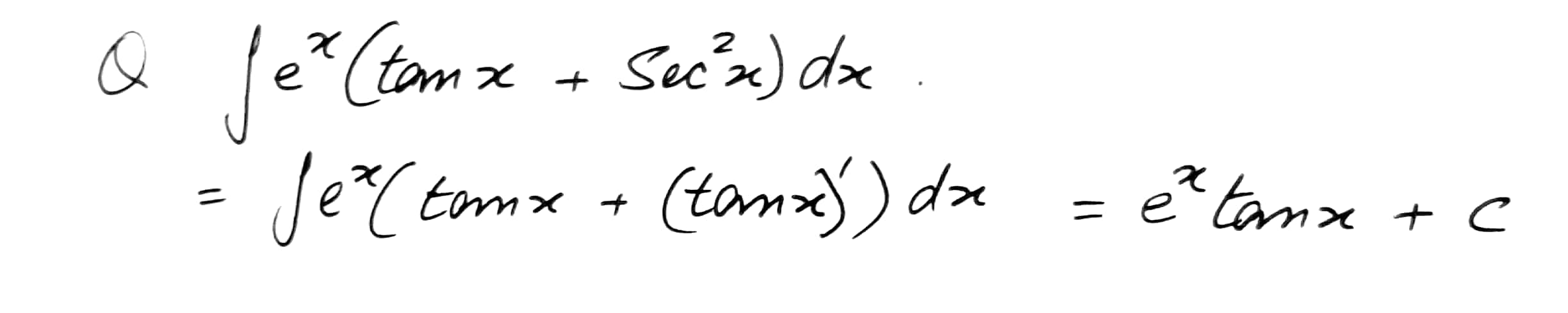
Mathematics Integration Level: Misc Level
Special type
Rule to evaluate
or
Where k is a real number (positive, negative or zero)
(i) Divide the numerator and denominator by x2
(ii) For x2 + A in the numerator put x - A/x =t as substitution . And for x2 - A in the numerator, put x + A/x = t

Mathematics Integration Level: Misc Level
Special type
Rule to evaluate
or
Where k is a real number (positive, negative or zero)
(i) Divide the numerator and denominator by x2
(ii) For x2 + A in the numerator put x - A/x =t as substitution . And for x2 - A in the numerator, put x + A/x = t

